Present Value of an Annuity; Amortization (3.4) In this section,
11 Slides69.50 KB

Present Value of an Annuity; Amortization (3.4) In this section, we will address the problem of determining the amount that should be deposited into an account now at a given interest rate in order to be able to withdraw equal amounts from the account in the future until no money remains in the account. Here is an example: How much money must you deposit now at 6% interest compounded quarterly in order to be able to withdraw 3,000 at the end of each quarter year for two years?

Derivation of formula We begin by solving for P in the compound interest formula: n A P 1 i P A(1 i ) n
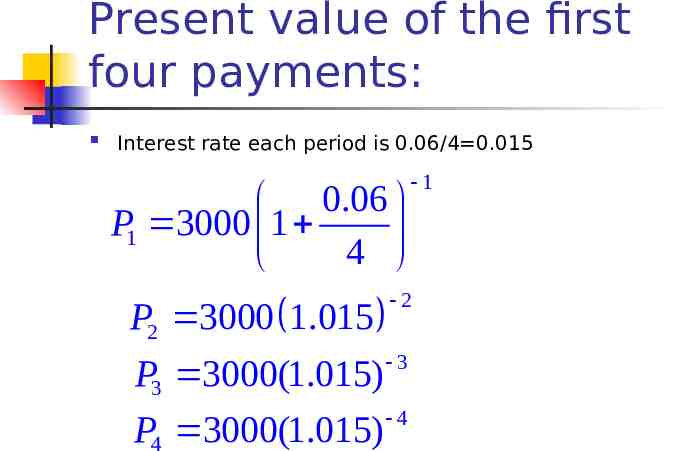
Present value of the first four payments: Interest rate each period is 0.06/4 0.015 0.06 P1 3000 1 4 1 2 P2 3000 1.015 3 P3 3000(1.015) P4 3000(1.015) 4

Derivation of short cut formula We could proceed to calculate the next four payments and then simply find the total of the 8 payments (there are 8 payments since there will be 8 total withdrawals – 2 years x four withdrawals per year 8 total withdrawals). This method is tedious and time consuming so we seek a short cut method.
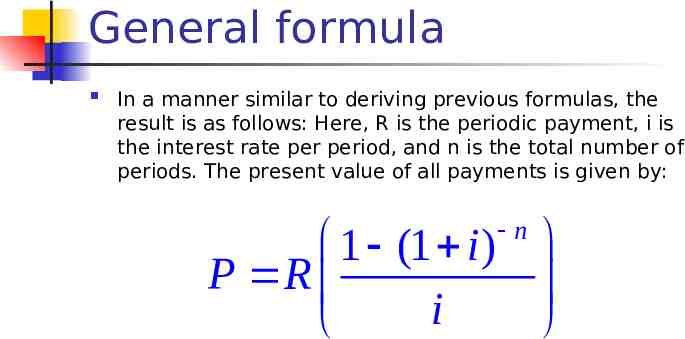
General formula In a manner similar to deriving previous formulas, the result is as follows: Here, R is the periodic payment, i is the interest rate per period, and n is the total number of periods. The present value of all payments is given by: n 1 (1 i ) P R i

Back to our original problem: How much money must you deposit now at 6% interest compounded quarterly in order to be able to withdraw 3,000 at the end of each quarter year for two years? Solution: R 3000, i 0.06/4 0.015, n 8 1 (1 i ) n P R i 1 (1.015) 8 P 3000 22, 457.78 0.015

Interest earned The present value of all payments is 22,457.78. The total amount of money withdrawn over two years is 3000(4)(2) 24,000. Thus, the accrued interest is the difference between the two amounts: 24,00022,457.78 1542.22.

Amortization Problem: A bank loans a customer 50,000 to purchase a house at 4.5% interest per year. The customer agrees to make monthly payments for the next 15 years for a total of 180 payments. How much should the monthly payment be if the debt is to be retired in 15 years? Solution: The bank has bought an annuity from the customer. This annuity pays the bank a PMT per month at 4.5% interest compounded monthly for 180 months.
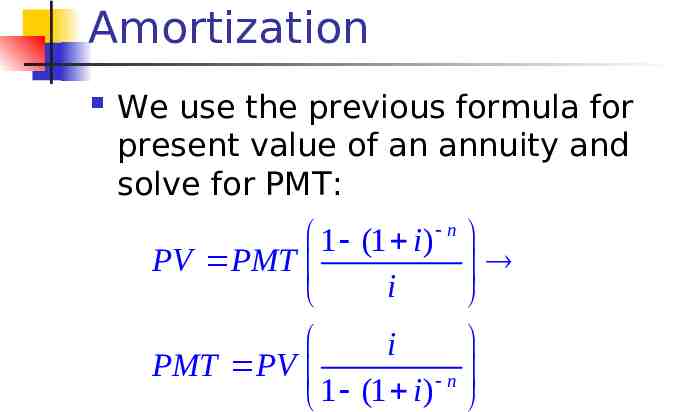
Amortization We use the previous formula for present value of an annuity and solve for PMT: 1 (1 i ) n PV PMT i i PMT PV n 1 (1 i )
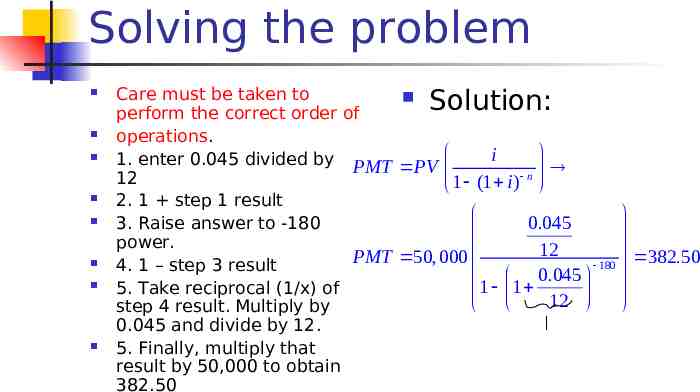
Solving the problem Solution: Care must be taken to perform the correct order of operations. i 1. enter 0.045 divided by PMT PV n 12 1 (1 i ) 2. 1 step 1 result 0.045 3. Raise answer to -180 power. 12 PMT 50, 000 4. 1 – step 3 result 0.045 180 5. Take reciprocal (1/x) of 1 1 12 step 4 result. Multiply by 0.045 and divide by 12. 5. Finally, multiply that result by 50,000 to obtain 382.50 382.50

Total amount of payments and interest paid If the customer makes a monthly payment of 382.50 to the bank for 180 payments, then the total amount paid to the bank is the product of 382.50 and 180 68,850. Thus, the interest earned by the bank is the difference between 68,850 and 50,000 (original loan) 18,850.






